How to estimate the depth of a subway station, to which you are accessing via an escalator? It happens that even to answer this question the knowledge of mathematics can be very useful. And in particular, of trigonometry.
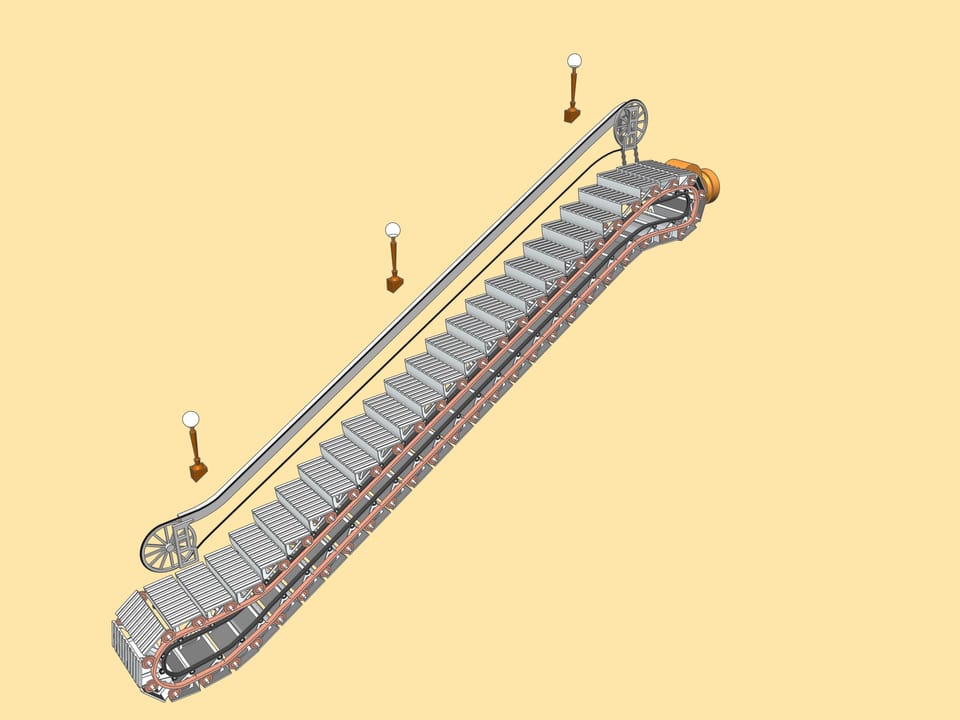
The escalator of the metropolitan railway... How many things are hidden behind these words for a curious observer. A huge machine in constant motion, a “living scale” ...
All began in the late nineteenth century, when the American manufacturer Jesse W. Reno (1861-1947) patented the first “living scale.” In its construction instead of the steps fixed to an “infinite” tape there were cylinders. But the first public escalator was made, according to its inventor, Charles D. Seeberger (1857-1931), from “Otis” industry and was exhibited at the Paris Exposition of 1900. This escalator had horizontal steps, coming out from under a fence on a platform of entry and disappeared under the same fence on another platform. However, this mechanism gave a lot of problems. In 1921 both ideas – that of horizontal steps and of cylinders — were put together to build a new model which, from that moment on, was always used.
When in the thirties the design of the Moscow subway started, the engineers tried to make use of foreign experience. However, both the cost and time required for its implementation by foreign companies were so large that the idea was abandoned. At that time the head of the London branch of the company “Otis” wrote to the representative of the Council of Moscow: “Your specialists are capable men. But the escalator is a very complicated affair, and you will not thrash out this problem. Even for us, with our capabilities and decades of experience, to run the project with those deadlines is impossible. As a friend of the Soviet Union, it is my duty to warn you that the date on which the subway will start can be not respected.” Yet, the Soviet engineers and scientists were able to solve this very special problem, and in February 1935 an escalator began to carry passengers to the subway station in Moscow.
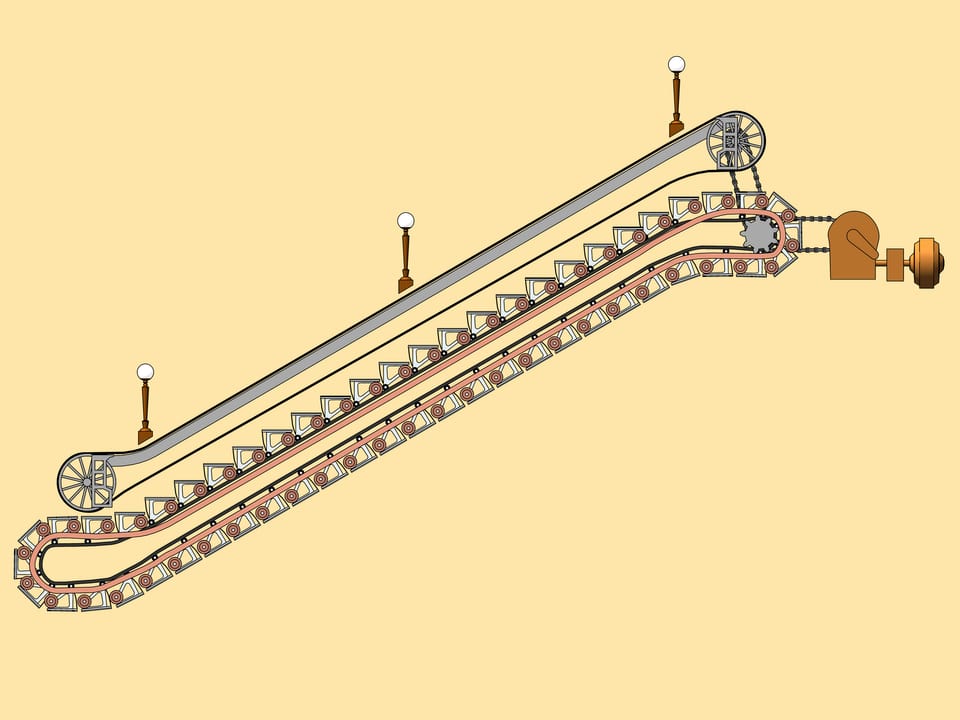
One of the important elements of the escalator is the step. It has four rollers, two large and two small. Both large and small rollers roll along their own tracks.
When the escalators were designed, even the choice of materials for the rollers was a very important and difficult problem. The Moscow Metro is open from 6 am until about one o’clock at night. That is, more than 19 hours — that is, more than 68000 seconds per day. The minimum speed of operation of a sliding scale is 0.75 m / s, which means that each step runs 50 km in one day. And so, tirelessly, day after day, over a year more than 18 thousand kilometers! Can you imagine of which kind of material the rollers should be made, to hold, without regular repairs and replacements, a fairly high amount of passengers that move on the steps. And this is just one detail and just one of all problems that the Soviet engineers had to solve, and of such problems and there were thousands.
Here is how the scheme of an escalator looks like. If we observe it in profile, we see that the mutual position of the rails of large rollers and of those of small rollers defines the fundamental property of the escalator: in the upper part of the “living scale”, on which the passengers stay, the steps are always horizontal. But at the bottom the steps are reversed and become parallel to the rails, saving space in the tunnel where they slide.
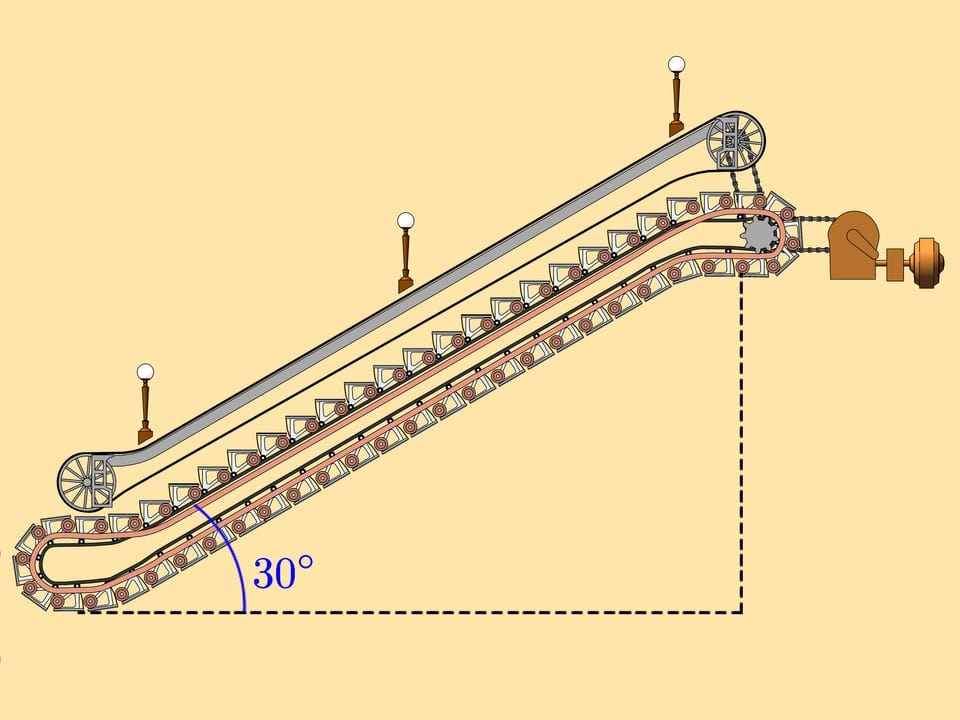
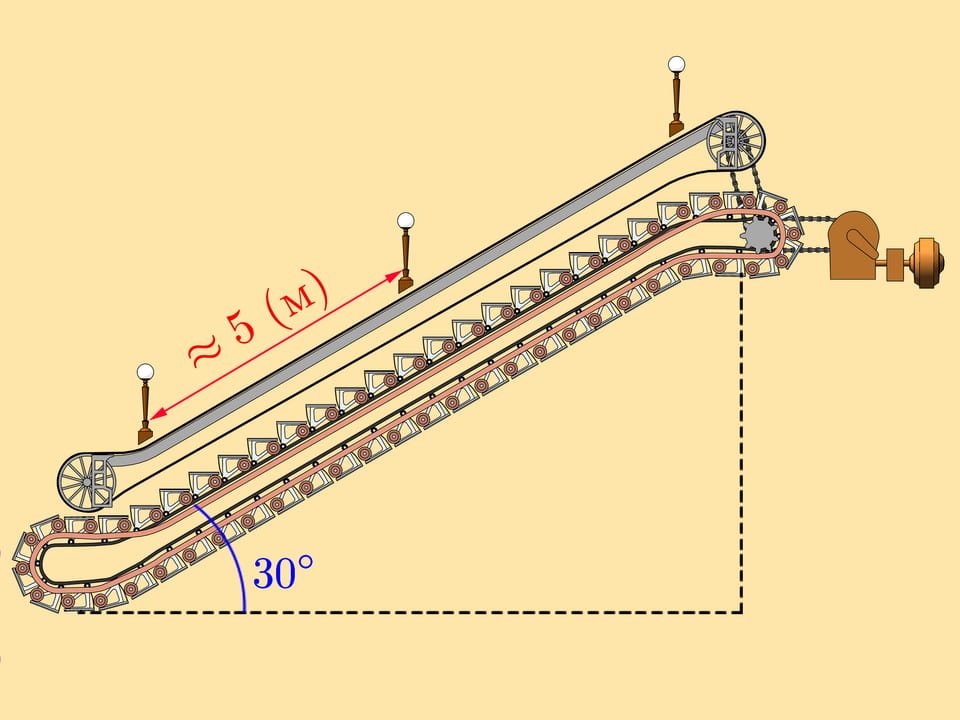
But let us come back to our question about the depth to which we are carried by the escalator. The surprising fact is that all Russian escalators, from the first ones up to the present days, are inclined by 30 degrees with respect to the horizontal!
Let us mentally construct an escalator on a right triangle. The length of its hypotenuse is the length of the escalator, while the length of the shorter cathetus is approximately equal to the depth of the station to which this staircase leads.
But how to calculate the length of the escalator while you are going on it? You could measure the time, but then for the calculation of the course you should know exactly the speed of the motion. But the speed can range from .75 m / s to 1 m / s and the error — a fourth — is quite large.
You could calculate the measures of a step, but in this case to know how many there are on the hypotenuse, while moving on the escalator, is complicated ...
What we can still use? Going down or going up an escalator, we continually meet lampposts! The distance between them is not fixed, but according to government rules, the tunnel lighting must have a certain intensity. And in total we obtain that the lampposts are located at about five meters one from the other.
Going down the escalator, we can count the number of lampposts. What should we do then, to calculate the length of the hypotenuse?
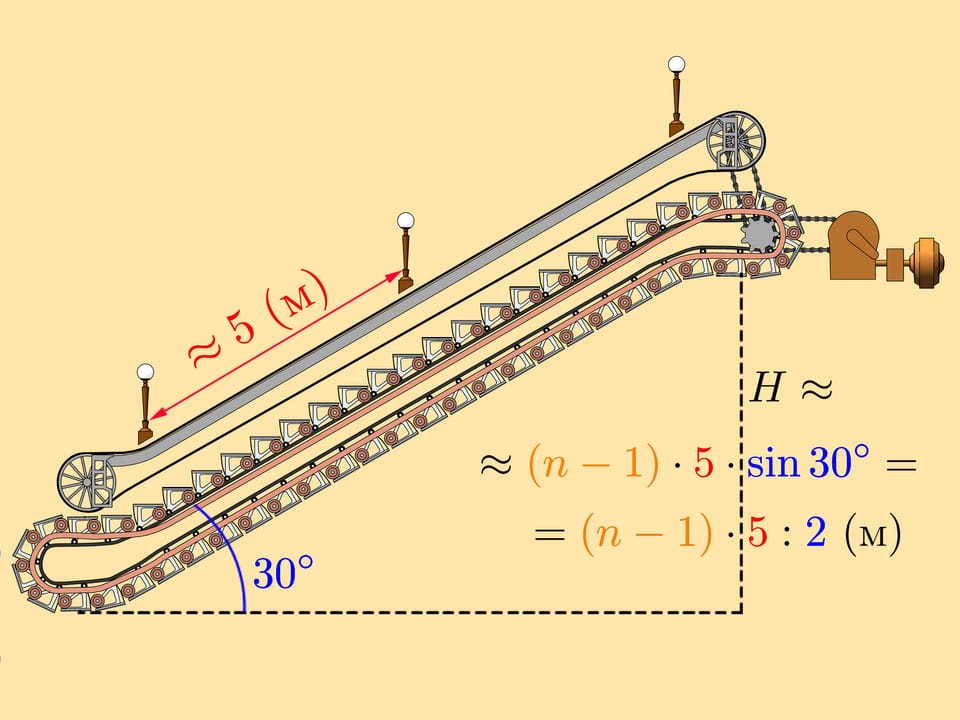
Do not rush to multiply by 5. For the calculation of the length we do not need the number of lamps, but the amount of distances between them! From the number of lamps we must subtract 1, then we can multiply by 5 and by the sine of 30°.
The great thing at this point is that the sine of 30 degrees is equal to ½, and this number is easy to count in your head! So the formula obtained for the depth of a station is very easy both to remember and to calculate.